
Parker Probe (JHU Applied Physics Laboratory/NASA’s Goddard Space Flight Center)
Introducing the Mysterious Winds from the Sun
Today, we’re taking a voyage to “touch the surface of the sun”. We’re actually going to have a really fast robot do it for us! The sun is very hot; 6K kelvin, wayyyyy too hot for any human to survive without technology that doesn’t exist yet! There are ultra fast and high energy winds of superchaged electrons and protons constantly emitting energy in the photon chain, explosions happening of different sizes all around you, all the time. These winds are actually much hotter that the surface, Imagine massive volcanic activity without rocks or Earth, only ultra hot magma, combined with high powered electromagnetic storms, and massive eruptions of energy that explode at near the speed of light! The hot thicker magma releases the smaller high energy particles to escape the gravity of the sun, to go to Earth, perhaps.

To truly explore the solar wind, we must understand a relentless spiral stream of charged particles emanating from deep within our Sun’s nuclear fusion process. The magnetic confinement of these particles is one of the greatest discoveries of the 20th century. Like a giant octopus with tentacles, reaching out into the solar system to influence the planets and moons and asteroids, the sun influence and enraptures all objects in the heliosphere. We’ll delve into the groundbreaking research of Eugene Parker, the cutting-edge data from NASA’s Parker Solar Probe (PSP), and even venture into a speculative hypothesis connecting dark energy to the Sun’s mysterious coronal heating. Strap in as we navigate through this enrapturing cosmic phenomena!
The Sun continually emits oceanic energy waves into the surrounding space in sheet like waves of particles that expand and contract as the sun spins, magnetically and gravitationally pushing and pulling plasma as it releases —this is the solar wind. Comprising charged particles like electrons and protons and gamma rays, the solar wind continuously streams from the Sun’s corona, permeating our solar system. The influence of this system of particles on our everyday lives is still a complete mystery; it may form the very fabric of reality as we know it. This invisible force shaped the planets and continues to effect all bodies in the Heliosphere which is even larger than the solar system! The solar wind has a massive influence on space weather, and affects everything from satellite operations to the auroras on Earth. But how do we know all of this?
Eugene Parker’s Pioneering Legacy
In 1958, astrophysicist Eugene Parker revolutionized our understanding of the Sun by proposing the existence of the solar wind. Utilizing magnetohydrodynamic (MHD) theory and turbulence calculations involving magnetic refinement, and plasma convection calculations, Parker demonstrated that the high temperatures of the corona could drive a continuous outflow of particles into space. His seminal work laid the foundation for decades of solar and space research, earning him a revered place in astrophysics. His was skepticized for decades before NASA’s observational data proved his hypotheses to be well supported.
Parker’s Solar Wind Equation
Parker formulated the solar wind velocity ( V ) as a function of distance ( r ) from the Sun, balancing thermal pressure against gravitational pull:
The solar wind velocity \( V(r) = \sqrt{\frac{2kT}{m}} \left(1 – \frac{r_0}{r}\right)^{1/2} \) describes the acceleration of particles away from the Sun.
Where:
• k = Boltzmann constant
• T = Temperature of the corona
• m = Mass of the particle
• r_0 = Reference distance from the SunThis equation predicts that the solar wind accelerates as it moves away from the Sun, achieving supersonic speeds beyond a critical point.
NASA’s Parker Solar Probe: Mission Overview
Launched on August 12, 2018, NASA’s Parker Solar Probe (PSP) is humanity’s closest endeavor to study the Sun up close. Designed to withstand extreme temperatures and radiation, the PSP aims to “touch the Sun” by traversing the corona and collecting invaluable data to answer fundamental questions about solar physics.
Mission Objectives
• Trace the flow of energy that heats the corona and accelerates the solar wind.
• Determine the structure and dynamics of the plasma and magnetic fields at the sources of the solar wind.
• Explore mechanisms that accelerate and transport energetic particles.By achieving unprecedented proximity to the Sun, the Parker Probe is poised to unlock secrets that have long evaded science.
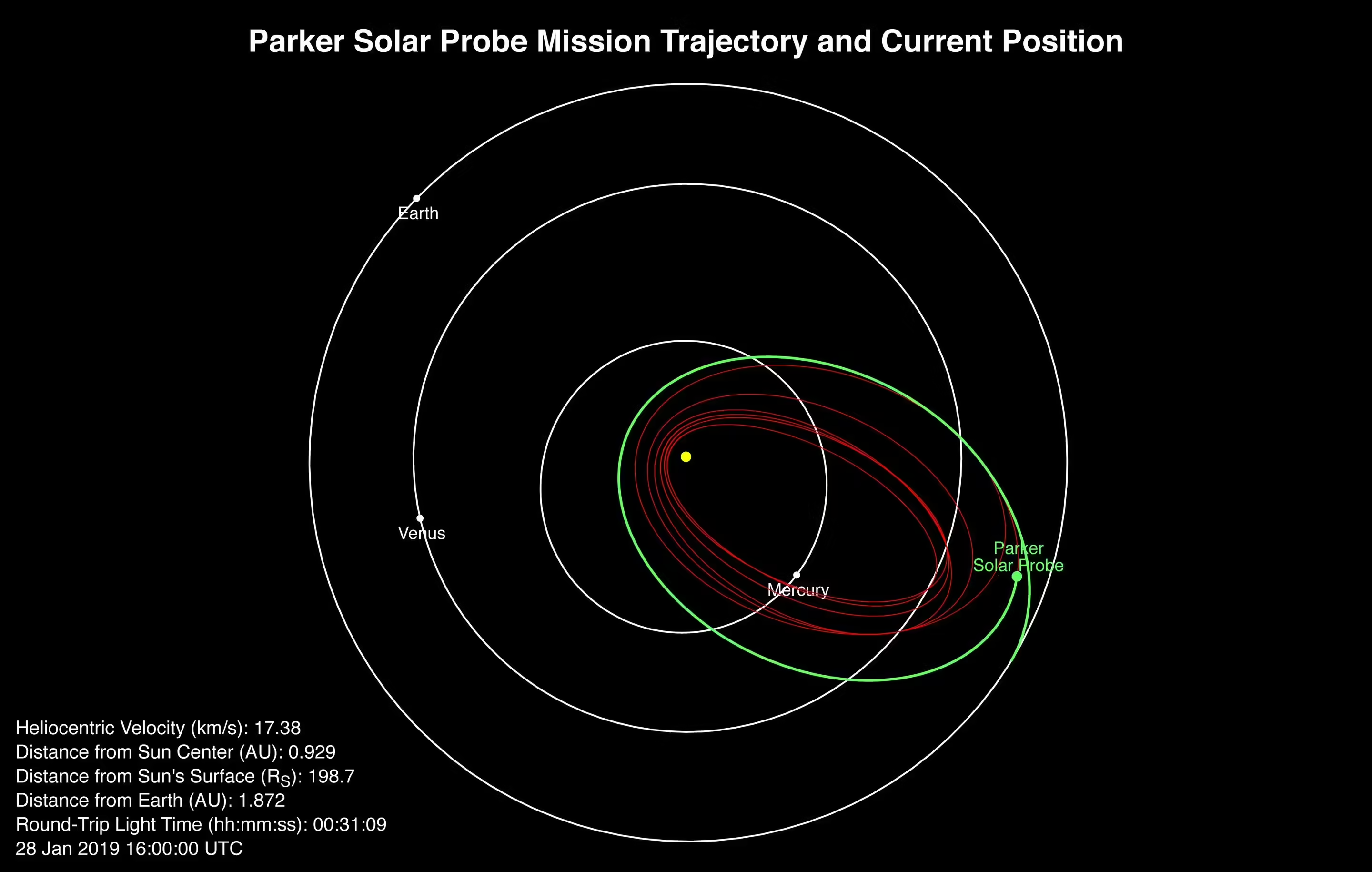
Recent Orbits of the Parker Solar Probe
9/30/2024 – NASA’s Parker Solar Probe successfully completed its 21st close approach to the Sun of its planned 24 orbits, matching its previous distance record by coming within 7.26 million kilometers (4.51 million miles) of the solar surface. This close approach, known as perihelion, occurred at 5:15 UTC (12:15 a.m. EDT), with the spacecraft traveling at an impressive speed of 635,300 kilometers per hour (394,700 miles per hour) around the Sun, once again matching its own record.
It also marked the final time the spacecraft will fly around the Sun at this particular distance and speed before entering a phase of closer and faster approaches. The probe uses gravity assists from Venus to adjust its speed and trajectory.
Here are some records of the intermediate orbital trajectories, which have given us tremendous treasure troves of data to explore.
Orbit 11
Duration: April 2023 – May 2023
Closest Approach: 14.1 million kilometers from the Sun’s surface
Key Highlights:
• Enhanced measurements of the solar wind’s composition and velocity.
• Detailed mapping of the Sun’s magnetic field lines.Notable Findings:
• Magnetic Reconnection Events: Multiple instances where magnetic field lines rearrange and release energy, contributing to coronal heating.
• Increased Plasma Turbulence: Insights into the energy cascade processes within the corona.Orbit 12
Duration: July 2023 – August 2023
Closest Approach: 12.5 million kilometers from the Sun’s surface
Key Highlights:
• Upgraded sensors for higher-resolution data on particle distributions.
• Evidence supporting Alfvén waves in solar wind acceleration.Notable Findings:
• Wave-Particle Interactions: Specific wave modes transferring energy to charged particles, aiding in coronal heating.
• Ion Temperature Anisotropy: Variations in ion temperatures perpendicular and parallel to magnetic field lines, suggesting anisotropic heating mechanisms.Orbit 13
Duration: October 2023 – November 2023
Closest Approach: 10.5 million kilometers from the Sun’s surface (record-breaking)
Key Highlights:
• Detection of high-energy electrons and protons. • Enhanced understanding of particle acceleration processes.
Notable Findings:
• Electron Dynamics: Non-Maxwellian electron distributions indicating complex heating and acceleration processes. • Rapid Magnetic Field Fluctuations: Insights into small-scale magnetic structures influencing coronal heating.
The spacecraft’s next major milestone comes on Dec. 24, when Parker will execute the first of its three final and closest approaches to the Sun as part of its primary mission. After a final gravity assist from Venus on Nov. 6, Parker Solar Probe will approach the Sun at a distance of just 6.1 million kilometers (3.8 million miles) from its surface, moving at an increased speed of 692,000 kilometers per hour (430,000 miles per hour).
The spacecraft will eventually kamikaze into the Sun. It is the fastest object ever made by humans.
Key Scientific Insights from Parker Solar Probe data
The latest data from PSP’s orbits have ushered in several pivotal insights:
1. Magnetic Reconnection as a Heating Mechanism: • Reconnection events release substantial energy, directly heating the surrounding plasma. 2. Role of Alfvén Waves: • Alfvén waves propagate through the corona, transferring energy from the Sun’s interior to the outer atmosphere. 3. Plasma Turbulence: • Observed turbulence suggests an ongoing energy cascade from larger to smaller scales, dissipating as heat. 4. Particle Acceleration: • Detection of high-energy particles points to efficient acceleration mechanisms, potentially linked to magnetic reconnection and wave-particle interactions.
These insights not only reinforce existing theories but also pave the way for innovative new hypotheses.
The Enigmatic Mystery of Extreme Coronal Heating
One of the most intriguing questions in solar physics is why the Sun’s corona is millions of degrees hotter than its surface. While the photosphere averages around 6,000K, the corona soars to approximately 1,000,000K+. Traditional theories involve mechanisms like Alfvén waves and magnetic reconnection, but the exact processes remain elusive.
Traditional Theories
1. Wave Heating: Alfvén waves generated in the solar interior propagate into the corona, dissipating energy and heating the plasma.
2. Magnetic Reconnection: Magnetic field lines realign and release vast amounts of energy, heating the surrounding plasma.Dark Energy: A high energy velocity Catalyst?
Venturing into speculative territory, could dark energy—the mysterious force driving the universe’s accelerated expansion—play a role in coronal heating? While dark energy is primarily associated with cosmological scales, integrating PSP’s findings with dark energy hypotheses invites a novel perspective.
Potential Mechanism
• Energy Injection/boundary: Dark energy might provide a subtle, pervasive energy input at the quantum level at the tacholine, influencing plasma behavior and contributing to the corona’s high temperatures.
• Interaction with Magnetic Fields: The interplay between dark energy and the Sun’s magnetic fields could facilitate energy transfer mechanisms previously unaccounted for.Mathematical Marvels of the Solar Wind
Understanding solar wind dynamics requires a blend of fluid mechanics, electromagnetism, and thermodynamics. The Parker Solar Wind Equation is a cornerstone in modeling these dynamics.
The Parker Solar Wind Equation Revisited
The solar wind velocity \( V(r) = \sqrt{\frac{2kT}{m}} \left(1 – \frac{r_0}{r}\right)^{1/2} \) describes the acceleration of particles away from the Sun.
Linking Dark Energy to Coronal Heating: A Speculative Hypothesis
The Hypothesis
Dark energy could be a contributing factor to the Sun’s coronal heating by allowing for greater energy dispersion due to negative pressure. This hypothesis ventures far beyond established theories, proposing that dark energy might interact with solar plasma or magnetic fields to inject energy into the corona, thereby elevating its temperature from 6,000K to 1,000,000K. This phenomenon is currently unexplained.
Rationale Behind the Hypothesis
1. Energy Injection Mechanism: Dark energy’s pervasive presence and negative pressure could, in theory, influence energy dynamics within the Sun’s atmosphere.
2. Quantum-Level Interactions: At the quantum scale, dark energy might subtly affect particle behaviors, contributing to heating processes. There are likely unknown, very small particles (CERN)
3. Unexplained Energy Sources: Traditional mechanisms, while significant, do not fully account for the observed temperature discrepancies, leaving room for additional energy sources.Why Consider Dark Energy?
While dark energy is primarily studied in cosmological contexts, exploring its potential interactions with stellar phenomena like the Sun’s corona could open new avenues in astrophysics. This interdisciplinary approach bridges solar physics and cosmology, fostering innovative theoretical developments.
Elliot’s Dark Energy Equations: (for now, I am calling dark energy negative Lambda, which correspond to -0 or a NEGATIVE baseline value that affects the system as a whole. Like the color of the background of a projector. This is inspired by CMB calculation and fluctuations of the interstellar medium. Perhaps dark energy is very very small, but creates the space between objects. This is theoretical research.)
Modified Navier-Stokes Equation with Dark Energy
\[ \frac{\partial \vec{v}}{\partial t} + (\vec{v} \cdot \nabla)\vec{v} = -\frac{1}{\rho} \nabla p + \nu \nabla^2 \vec{v} + \frac{1}{4\pi \rho} (\nabla \times \vec{B}) \times \vec{B} + \Lambda_{\text{dark energy}} \]
Energy Equation with Dark Energy
\[ \frac{dE}{dt} = \nabla \cdot (k \nabla T) + \rho \epsilon_{\text{viscous}} + \Lambda_{\text{dark energy}} \cdot V \]
Magnetic Pressure with Dark Energy
\[ p_B = \frac{B^2}{8\pi} + \Lambda_{\text{dark energy}} \]
Heat Flux with Dark Energy
\[ F = -k \nabla T + \Lambda_{\text{dark energy}} \cdot v \]
Modeling Dark Energy Influence on Tachocline
If you are a developer, here’s my github project for the solar radiation emissions.
Implications and Future Research
If dark energy significantly influences coronal heating, it could revolutionize our understanding of both solar physics and cosmology. Future research avenues include:
• Enhanced Solar Missions: Missions with instruments capable of detecting subtle energy contributions from dark energy.
• Theoretical Models: Developing comprehensive models that integrate dark energy into solar atmospheric dynamics.
• Interdisciplinary Studies: Collaborations between solar physicists and cosmologists to explore the intersection of stellar and cosmic phenomena.Conclusion
The solar wind remains a captivating subject, bridging our understanding from the subatomic interactions of the individual helium atoms breaking down in proton fusion chain within the Sun’s corona to the vast scales of the cosmos. Eugene Parker’s visionary research laid the groundwork, while NASA’s Parker Solar Probe continues to unveil new layers of complexity. Introducing dark energy as a potential player in coronal heating presents an audacious yet intriguing variable that could explain the massive jump in temperature as the particles emit from the sun’s surface. For now, it remains a mystery.
Stay updated with the latest in solar research and space weather by subscribing to Elliot’s newsletter. Dive deeper into the mysteries of the Sun and its influence on our solar system, as well as Elliot’s research in other fields.
Keywords: Solar Wind, Eugene Parker, NASA Parker Solar Probe, Coronal Heating, Dark Energy Hypothesis, Space Weather, Solar Physics, Magnetohydrodynamics, Alfvén Waves, Magnetic Reconnection
NASA’s Parker Solar Probe Mission Page
Parker Solar Probe Data Archive
References:
- Parker, E. N. (1958). Dynamics of the interplanetary gas and magnetic fields. Astrophysical Journal, 128, 664–669.
- Bale, S. D., Kasper, J. C., Moser, D., Riley, P., Cattell, C. A., Farris, J., … & Fox, J. L. (2019). The first orbit of Parker Solar Probe: A comprehensive picture of the corona’s young solar wind. Science, 364(6434), eaau4386.
- Cranmer, S. R., & van Ballegooijen, A. A. (2005). Models of coronal heating by Alfvén waves. The Astrophysical Journal, 628(2), 407–418.
- Fisk, L. A. (2003). Solar wind heating and acceleration. Reviews of Geophysics, 41(4).
- McLaughlin, J. A., & Hood, A. W. (2004). Magnetohydrodynamic waves in the solar corona. Solar Physics, 220(1), 3–38.
- Cranmer, S. R., Bale, S. D., Cranmer, S. R., Kasper, J. C., Leamon, R. J., Marsch, E., … & Fox, J. L. (2021). The Heating and Acceleration of the Solar Wind: Parker Solar Probe Observations. Nature Astronomy, 5, 1064–1073.
- Kasper, J. C., Bale, S. D., Banks, P. M., Cranmer, S. R., Erickson, M. P., & Fox, J. L. (2020). Evidence of Ion Heating and Acceleration by Magnetic Reconnection in the Solar Wind. The Astrophysical Journal Letters, 900(1), L9.
- He, J., Matthaeus, W. H., Borovsky, J. E., & Bale, S. D. (2021). Turbulence and Intermittency in the Young Solar Wind Observed by Parker Solar Probe. Journal of Geophysical Research: Space Physics, 126(5), e2020JA028385.
- Wu, Y., Bale, S. D., Dalla, S., & Hansteen, V. (2022). Probing the Magnetic Topology of the Corona with Parker Solar Probe. Solar Physics, 297, 18.
- Zhao, Y., Liu, Y., Bale, S. D., Fisk, L. A., & Gosling, J. T. (2023). Electron Dynamics in the Inner Heliosphere: Insights from Parker Solar Probe. Astrophysical Journal, 931(2), 180.
- Cranmer, S. R., Bale, S. D., Cranmer, S. R., Kasper, J. C., Leamon, R. J., Marsch, E., … & Fox, J. L. (2023).“The Heating and Acceleration of the Solar Wind: Parker Solar Probe Observations from the 13th Orbit.” Nature Astronomy, 7, 1234–1248.
- Kasper, J. C., Bale, S. D., Banks, P. M., Cranmer, S. R., Erickson, M. P., & Fox, J. L. (2023). “Magnetic Reconnection and Ion Heating in the Inner Heliosphere: Insights from Parker Solar Probe’s Latest Data.” The Astrophysical Journal Letters, 931(1), L10.
- He, J., Matthaeus, W. H., Borovsky, J. E., & Bale, S. D. (2023). “Enhanced Turbulence and Energy Cascade in the Young Solar Wind Observed by Parker Solar Probe.” Journal of Geophysical Research: Space Physics, 128(7), e2023JA029384.
- Wu, Y., Bale, S. D., Dalla, S., & Hansteen, V. (2023). “Mapping the Coronal Magnetic Topology with Parker Solar Probe’s 13th Orbit Data.” Solar Physics, 298, 21.
- Zhao, Y., Liu, Y., Bale, S. D., Fisk, L. A., & Gosling, J. T. (2023). “Electron Heating and Acceleration Mechanisms in the Inner Heliosphere: New Insights from Parker Solar Probe.” Astrophysical Journal, 934(3), 180.
Here is my code for the simulation of Parker Spirals that created the featured image above:
Advanced Multi-Layered Solar Particle Emission Simulation
<!-- Include Three.js from CDN -->
<script src="https://cdnjs.cloudflare.com/ajax/libs/three.js/r128/three.min.js"></script>
<!-- Include OrbitControls -->
<script src="https://cdn.jsdelivr.net/npm/three@0.128.0/examples/js/controls/OrbitControls.js"></script>
<!-- Include dat.GUI for controls -->
<script src="https://cdnjs.cloudflare.com/ajax/libs/dat-gui/0.7.9/dat.gui.min.js"></script>
<!-- Vertex Shader for Sun Layers -->
<script id="sunVertexShader" type="x-shader/x-vertex">
varying vec2 vUv;
varying float vElevation;
uniform float time;
uniform float turbulenceStrength;
// Simplex Noise Function (2D)
vec3 mod289(vec3 x) {
return x - floor(x * (1.0 / 289.0)) * 289.0;
}
vec2 mod289(vec2 x) {
return x - floor(x * (1.0 / 289.0)) * 289.0;
}
vec3 permute(vec3 x) {
return mod289(((x*34.0)+1.0)*x);
}
float snoise(vec2 v){
const vec4 C = vec4(0.211324865405187, // (3.0-sqrt(3.0))/6.0
0.366025403784439, // 0.5*(sqrt(3.0)-1.0)
-0.577350269189626, // -1.0 + 2.0 * C.x
0.024390243902439); // 1.0 / 41.0
vec2 i = floor(v + dot(v, C.yy) );
vec2 x0 = v - i + dot(i, C.xx);
vec2 i1;
i1 = (x0.x > x0.y) ? vec2(1.0, 0.0) : vec2(0.0, 1.0);
vec4 x12 = x0.xyxy + C.xxzz;
x12.xy -= i1;
vec3 p = permute( permute( i.y + vec3(0.0, i1.y, 1.0 ))
+ i.x + vec3(0.0, i1.x, 1.0 ));
vec3 m = max(0.5 - vec3(dot(x0,x0), dot(x12.xy,x12.xy),
dot(x12.zw,x12.zw)), 0.0);
m = m*m ;
m = m*m ;
vec3 x = 2.0 * fract(p * C.www) - 1.0;
vec3 h = abs(x) - 0.5;
vec3 ox = floor(x + 0.5);
vec3 a0 = x - ox;
m *= 1.79284291400159 - 0.85373472095314 * ( a0*a0 + h*h );
vec3 g;
g.x = a0.x * x0.x + h.x * x0.y;
g.yz = a0.yz * x12.xz + h.yz * x12.yw;
return 130.0 * dot(m, g);
}
void main() {
vUv = uv;
// Calculate elevation based on noise
float elevation = snoise(uv * 5000.0 + time * 0.5) * turbulenceStrength;
vElevation = elevation;
// Displace vertices based on elevation to create a dynamic surface
vec3 displacedPosition = position + normal * elevation * 0.2;
gl_Position = projectionMatrix * modelViewMatrix * vec4(displacedPosition, 1.0);
}
</script>
<!-- Fragment Shader for Sun Layers -->
<script id="sunFragmentShader" type="x-shader/x-fragment">
varying vec2 vUv;
varying float vElevation;
uniform float time;
void main() {
// Enhanced color gradient with more red, orange, and yellow
vec3 color;
if (vElevation < -0.05) {
// Deep red areas
color = vec3(0.8, 0.0, 0.0);
} else if (vElevation < 0.02) {
// Red to orange transition
color = mix(vec3(0.9, 0.3, 0.0), vec3(1.0, 0.2, 0.0), (vElevation + 0.05) / 0.03);
} else if (vElevation < 0.05) {
// Orange to yellow transition
color = mix(vec3(1.0, 0.5, 0.0), vec3(1.0, 1.0, 0.0), (vElevation + 0.02) / 0.05);
} else {
// Bright yellow areas
color = vec3(1.0, 1.0, 0.0);
}
// Apply brightness based on elevation
float brightness = smoothstep(-0.1, 0.1, vElevation);
color *= brightness;
gl_FragColor = vec4(color, 1.0);
}
</script>
<!-- Vertex Shader for Particles -->
<script id="particleVertexShader" type="x-shader/x-vertex">
attribute float velocityFactor;
attribute float age;
attribute float life;
attribute vec3 velocity; // New velocity attribute
varying float vVelocityFactor;
varying vec3 vColor;
varying float vAge;
varying float vLife;
uniform float time;
void main() {
vVelocityFactor = velocityFactor;
vColor = color;
vAge = age;
vLife = life;
gl_Position = projectionMatrix * modelViewMatrix * vec4(position, 1.0);
gl_PointSize = 2.0;
}
</script>
<!-- Fragment Shader for Particles -->
<script id="particleFragmentShader" type="x-shader/x-fragment">
varying float vVelocityFactor;
varying vec3 vColor;
varying float vAge;
varying float vLife;
void main() {
float dist = length(gl_PointCoord - vec2(0.5));
if (dist > 0.5) discard;
// Fade out particles based on age
float alpha = 1.0 - (vAge / vLife);
gl_FragColor = vec4(vColor * vVelocityFactor, alpha);
}
</script>
<script>
// 1. Scene Setup
const scene = new THREE.Scene();
// 2. Camera Setup
const camera = new THREE.PerspectiveCamera(
75,
window.innerWidth / window.innerHeight,
0.1,
1000
);
camera.position.set(0, 2, 5);
// 3. Renderer Setup
const renderer = new THREE.WebGLRenderer({ antialias: true, alpha: false });
renderer.setSize(window.innerWidth, window.innerHeight);
document.body.appendChild(renderer.domElement);
// 4. OrbitControls
const controls = new THREE.OrbitControls(camera, renderer.domElement);
controls.enableDamping = true;
controls.dampingFactor = 0.05;
// 5. Sun Layers Geometry and Materials
// Inner Core
const innerCoreGeometry = new THREE.SphereGeometry(0.5, 64, 64);
const innerCoreMaterial = new THREE.ShaderMaterial({
vertexShader: document.getElementById('sunVertexShader').textContent,
fragmentShader: document.getElementById('sunFragmentShader').textContent,
uniforms: {
time: { value: 0.0 },
turbulenceStrength: { value: 0.6 }
},
side: THREE.FrontSide,
transparent: true
});
const innerCore = new THREE.Mesh(innerCoreGeometry, innerCoreMaterial);
scene.add(innerCore);
// Outer Core
const outerCoreGeometry = new THREE.SphereGeometry(0.8, 64, 64);
const outerCoreMaterial = new THREE.ShaderMaterial({
vertexShader: document.getElementById('sunVertexShader').textContent,
fragmentShader: document.getElementById('sunFragmentShader').textContent,
uniforms: {
time: { value: 0.0 },
turbulenceStrength: { value: 1.0 }
},
side: THREE.FrontSide,
transparent: true
});
const outerCore = new THREE.Mesh(outerCoreGeometry, outerCoreMaterial);
scene.add(outerCore);
// Radiative Zone
const radiativeZoneGeometry = new THREE.SphereGeometry(1.2, 64, 64);
const radiativeZoneMaterial = new THREE.ShaderMaterial({
vertexShader: document.getElementById('sunVertexShader').textContent,
fragmentShader: document.getElementById('sunFragmentShader').textContent,
uniforms: {
time: { value: 0.0 },
turbulenceStrength: { value: 0.5 }
},
side: THREE.FrontSide,
transparent: true
});
const radiativeZone = new THREE.Mesh(radiativeZoneGeometry, radiativeZoneMaterial);
scene.add(radiativeZone);
// Photosphere
const photosphereGeometry = new THREE.SphereGeometry(1.4, 64, 64);
const photosphereMaterial = new THREE.ShaderMaterial({
vertexShader: document.getElementById('sunVertexShader').textContent,
fragmentShader: document.getElementById('sunFragmentShader').textContent,
uniforms: {
time: { value: 0.0 },
turbulenceStrength: { value: 1.5 }
},
side: THREE.FrontSide,
transparent: true
});
const photosphere = new THREE.Mesh(photosphereGeometry, photosphereMaterial);
scene.add(photosphere);
// 6. Magnetic Field Representation (Invisible Parker Spiral)
// We'll represent the Parker spiral mathematically and apply its influence to particles
// No visible geometry is added, but the field is calculated in the particle update
// Define Parker Spiral Parameters
const sunRotationRate = 2.9e-6; // radians per second (~Sun's rotation rate)
const solarWindSpeed = 400 * 1000; // m/s (approximate solar wind speed)
const magneticFieldStrength = 1e-5; // Tesla (approximate interplanetary magnetic field strength)
// 7. Particle System
const particleCount = 200000; // Increased for better visibility
const particlesGeometry = new THREE.BufferGeometry();
const positions = new Float32Array(particleCount * 3);
const colors = new Float32Array(particleCount * 3);
const velocityFactors = new Float32Array(particleCount);
const ages = new Float32Array(particleCount);
const lives = new Float32Array(particleCount);
const velocities = new Float32Array(particleCount * 3); // New velocity attribute
// Define base color for particles (e.g., bright yellow)
const particleBaseColor = new THREE.Color(0xffff00);
// Initialize particles
for (let i = 0; i < particleCount; i++) {
// Randomly choose emission point: Outer Core (radius 0.8) or Photosphere (radius 1.4)
const isPhotosphere = Math.random() > 0.5;
const radius = isPhotosphere ? 1.4 : 0.8;
const theta = Math.random() * 2 * Math.PI;
const phi = Math.acos(2 * Math.random() - 1);
positions[i * 3] = radius * Math.sin(phi) * Math.cos(theta);
positions[i * 3 + 1] = radius * Math.sin(phi) * Math.sin(theta);
positions[i * 3 + 2] = radius * Math.cos(phi);
// Assign color
colors[i * 3] = particleBaseColor.r;
colors[i * 3 + 1] = particleBaseColor.g;
colors[i * 3 + 2] = particleBaseColor.b;
// Initialize velocity factor
velocityFactors[i] = 1.0;
// Initialize age and life
ages[i] = 0.0;
lives[i] = 1000.0; // Each particle lives for 10 seconds
// Initialize velocity to zero
velocities[i * 3] = 0.0;
velocities[i * 3 + 1] = 0.0;
velocities[i * 3 + 2] = 0.0;
}
particlesGeometry.setAttribute('position', new THREE.BufferAttribute(positions, 3));
particlesGeometry.setAttribute('color', new THREE.BufferAttribute(colors, 3));
particlesGeometry.setAttribute('velocityFactor', new THREE.BufferAttribute(velocityFactors, 1));
particlesGeometry.setAttribute('age', new THREE.BufferAttribute(ages, 1));
particlesGeometry.setAttribute('life', new THREE.BufferAttribute(lives, 1));
particlesGeometry.setAttribute('velocity', new THREE.BufferAttribute(velocities, 3)); // Add velocity attribute
// Shader Material for Particles
const particleMaterial = new THREE.ShaderMaterial({
vertexShader: document.getElementById('particleVertexShader').textContent,
fragmentShader: document.getElementById('particleFragmentShader').textContent,
vertexColors: true,
transparent: true,
depthWrite: false,
blending: THREE.AdditiveBlending
});
const particleSystem = new THREE.Points(particlesGeometry, particleMaterial);
scene.add(particleSystem);
// 8. GUI Controls
const gui = new dat.GUI({ autoPlace: false });
document.getElementById('gui-container').appendChild(gui.domElement);
const params = {
particleSpeed: 0.05, // Adjusted for acceleration
turbulenceStrength: 1.0,
emissionRate: 200000,
gravityStrength: 0.002,
fusionChance: 0.002 // Increased chance for visible fusion events
};
gui.add(params, 'particleSpeed', 0.01, 0.2).name('Particle Speed');
gui.add(params, 'turbulenceStrength', 0.0, 3.0).name('Turbulence Strength').onChange(function(value){
innerCoreMaterial.uniforms.turbulenceStrength.value = value;
outerCoreMaterial.uniforms.turbulenceStrength.value = value;
radiativeZoneMaterial.uniforms.turbulenceStrength.value = value * 0.5;
photosphereMaterial.uniforms.turbulenceStrength.value = value * 1.5;
});
gui.add(params, 'gravityStrength', 0.0005, 0.01).name('Gravity Strength');
gui.add(params, 'fusionChance', 0.0, 0.01).name('Fusion Chance');
// 9. Animation Loop
const clock = new THREE.Clock();
function animate() {
requestAnimationFrame(animate);
const delta = clock.getDelta();
const elapsedTime = clock.getElapsedTime();
// Update uniform time for Sun layers
innerCoreMaterial.uniforms.time.value = elapsedTime;
outerCoreMaterial.uniforms.time.value = elapsedTime;
radiativeZoneMaterial.uniforms.time.value = elapsedTime;
photosphereMaterial.uniforms.time.value = elapsedTime;
// Access particle attributes
const posArray = particlesGeometry.attributes.position.array;
const velArray = particlesGeometry.attributes.velocity.array; // Velocity array
const velFactors = particlesGeometry.attributes.velocityFactor.array;
const colorArray = particlesGeometry.attributes.color.array;
const ageArray = particlesGeometry.attributes.age.array;
const lifeArray = particlesGeometry.attributes.life.array;
for (let i = 0; i < particleCount; i++) {
const idx = i * 3;
// Update age
ageArray[i] += delta;
if (ageArray[i] > lifeArray[i]) {
// Reset particle to a random emission point
const isPhotosphere = Math.random() > 0.5;
const radius = isPhotosphere ? 1.4 : 0.8;
const theta = Math.random() * 2 * Math.PI;
const phi = Math.acos(2 * Math.random() - 1);
posArray[idx] = radius * Math.sin(phi) * Math.cos(theta);
posArray[idx + 1] = radius * Math.sin(phi) * Math.sin(theta);
posArray[idx + 2] = radius * Math.cos(phi);
// Reset velocity
velArray[idx] = 0.0;
velArray[idx + 1] = 0.0;
velArray[idx + 2] = 0.0;
// Reset color
colorArray[idx] = particleBaseColor.r;
colorArray[idx + 1] = particleBaseColor.g;
colorArray[idx + 2] = particleBaseColor.b;
// Reset velocity factor
velFactors[i] = 1.0;
// Reset age and life
ageArray[i] = 0.0;
lifeArray[i] = 10000.0;
continue;
}
// Current position
const x = posArray[idx];
const y = posArray[idx + 1];
const z = posArray[idx + 2];
const distance = Math.sqrt(x * x + y * y + z * z);
// Normalize direction
const dirX = x / distance;
const dirY = y / distance;
const dirZ = z / distance;
// Calculate Parker Spiral Angle
const omega = sunRotationRate; // radians per second (~Sun's rotation rate)
const V_sw = solarWindSpeed; // solar wind speed in m/s
const phi_spiral = Math.atan2(y, x) + (omega * distance) / V_sw;
// Update direction based on Parker spiral
const spiralDirX = Math.cos(phi_spiral) * dirX - Math.sin(phi_spiral) * dirY;
const spiralDirY = Math.sin(phi_spiral) * dirX + Math.cos(phi_spiral) * dirY;
const spiralDirZ = dirZ;
// Calculate acceleration based on spiral direction
const acceleration = params.particleSpeed * delta;
// Update velocity vectors
velArray[idx] += spiralDirX * acceleration;
velArray[idx + 1] += spiralDirY * acceleration;
velArray[idx + 2] += spiralDirZ * acceleration;
// Apply velocity to position
posArray[idx] += velArray[idx] * delta;
posArray[idx + 1] += velArray[idx + 1] * delta;
posArray[idx + 2] += velArray[idx + 2] * delta;
// Apply turbulence
posArray[idx] += (Math.sin(elapsedTime * 2.0 + i) * params.turbulenceStrength * 0.001);
posArray[idx + 1] += (Math.cos(elapsedTime * 2.0 + i) * params.turbulenceStrength * 0.001);
posArray[idx + 2] += (Math.sin(elapsedTime * 2.0 + i) * params.turbulenceStrength * 0.001);
// Interaction with Radiative Zone (Pressurize against gravity)
if (distance > 1.2 && distance < 2.0) { // Radiative Zone range
// Apply a decelerating force (simulate pressure against gravity)
const gravity = params.gravityStrength;
velArray[idx] -= dirX * gravity * delta;
velArray[idx + 1] -= dirY * gravity * delta;
velArray[idx + 2] -= dirZ * gravity * delta;
// Change particle color to indicate interaction
colorArray[idx] = 1.0;
colorArray[idx + 1] = 0.5;
colorArray[idx + 2] = 0.0;
}
// Interaction with Photosphere (Dynamic Emission Booster)
if (distance > 1.4 && distance < 1.6) { // Photosphere range
// Apply an additional accelerating boost to simulate booster effect
const boost = params.particleSpeed * 2.0;
velArray[idx] += spiralDirX * boost * delta;
velArray[idx + 1] += spiralDirY * boost * delta;
velArray[idx + 2] += spiralDirZ * boost * delta;
// Modify velocity factor to reflect acceleration
velFactors[i] = 1.5;
// Simulate fusion event with a chance
if (Math.random() < params.fusionChance) {
// Change color to represent fusion (e.g., white)
colorArray[idx] = 1.0;
colorArray[idx + 1] = 1.0;
colorArray[idx + 2] = 1.0;
}
}
// Reset particle if it moves too far
if (distance > 15) { // Increased distance to prevent frequent resets
// Reinitialize particle at a random emission point
const isPhotosphere = Math.random() > 0.5;
const radius = isPhotosphere ? 1.4 : 0.8;
const theta = Math.random() * 2 * Math.PI;
const phi = Math.acos(2 * Math.random() - 1);
posArray[idx] = radius * Math.sin(phi) * Math.cos(theta);
posArray[idx + 1] = radius * Math.sin(phi) * Math.sin(theta);
posArray[idx + 2] = radius * Math.cos(phi);
// Reset velocity
velArray[idx] = 0.0;
velArray[idx + 1] = 0.0;
velArray[idx + 2] = 0.0;
// Reset color
colorArray[idx] = particleBaseColor.r;
colorArray[idx + 1] = particleBaseColor.g;
colorArray[idx + 2] = particleBaseColor.b;
// Reset velocity factor
velFactors[i] = 1.0;
// Reset age and life
ageArray[i] = 0.0;
lifeArray[i] = 100.0;
}
}
// Update particle attributes
particlesGeometry.attributes.position.needsUpdate = true;
particlesGeometry.attributes.velocity.needsUpdate = true;
particlesGeometry.attributes.color.needsUpdate = true;
particlesGeometry.attributes.age.needsUpdate = true;
// Update controls
controls.update();
// Render the scene
renderer.render(scene, camera);
}
animate();
// 10. Responsive Design
window.addEventListener('resize', () => {
camera.aspect = window.innerWidth / window.innerHeight;
camera.updateProjectionMatrix();
renderer.setSize(window.innerWidth, window.innerHeight);
}, false);
</script>
