Black Hole Projects
ExploreOver the next few months, the launch window to Mars will open as the planets align for travel. There will be a sweet spot where launch will be fastest; I am going to try to calculate this window!
For now, lets do some research on what the heck something like this even entails.
Let’s think about the orbits of the planets:
a. Earth’s Orbital Characteristics
- Semi-Major Axis (Average Distance from Sun): ~149.6 million km (1 Astronomical Unit, AU)
- Orbital Period: ~365.25 days
- Eccentricity: ~0.0167 (nearly circular)
- Orbital Speed: ~29.78 km/s
b. Mars’ Orbital Characteristics
- Semi-Major Axis: ~227.9 million km (~1.524 AU)
- Orbital Period: ~687 Earth days (~1.88 Earth years)
- Eccentricity: ~0.0934 (more elliptical than Earth)
- Orbital Speed: ~24.077 km/s
Hohmann Transfer Orbit
Considering this type of trajectory, the window opens when Mars is about 44 degrees ahead of Earth in its 2 dimensional orbit. This is currently the most efficient orbital maneuver for fuel conservation.

By Phoenix7777 – Own workData source: HORIZONS System, JPL, NASA, CC BY-SA 4.0, Link
It is important to keep in mind that these are two large objects with completely different gravity fields and atmospheres; in order to truly understand the amount of fuel and velocity required to get there faster we can use Hohmann’s calculations for changing orbital trajectories.
a. Delta-V Overview
Delta-V is a measure of the difference of velocities required to perform maneuvers in space; so both slowing down the spacecraft and accelerating it in space as it enters or leaves a planetary body’s gravity well.
b. Hohmann Transfer Delta-V
(note: if we could get the delta V up to 7(the velocity of the rocket as it leaves orbit), we could probably get to Mars in closer to 4 months.)
The Hohmann transfer orbit is the ellipse that connects the points in space, Earth at 0 degrees and Mars at 180 degrees, about the ellipse that has the sun at one focus. This maneuver is incredibly complex and notoriously difficult as evidenced by the simulation below; there is a complex maneuver required called aero-braking where the spacecraft uses friction and resistance of atmosphere by dipping into the atmosphere at the periapsis(low point) of its orbit to slow itself down. Some kind of manual braking is required by Mars’ lack of atmosphere; rocket propulsion will likely accompany SpaceX’s braking system.

First Missions
Mariner 4 was the first craft to reach Mars. The spacecraft launched on Nov. 28, 1964, and was the first to fly by the planet on July 14, 1965. It took 21 pictures; the first ever from deep space.
The Mars Global Surveyor was the first real spacecraft to test aero-braking. The mission is fascinating: The spacecraft was launched from a smaller Delta II rocket, necessitating restrictions in spacecraft weight. In order to achieve the near-circular orbit required for the mission while conserving propellant, the team designed a series of aerobraking maneuvers. Aero-braking had been successfully attempted by the Magellan mission at Venus, but the first complete test of the new procedure was to be carried out by MGS.[17]
Initially, MGS was placed in a highly elliptical orbit that took 45 hours to complete. The orbit had a periapsis of 262 km (163 mi) above the northern hemisphere, and an apoapsis of 54,026 km (33,570 mi) above the southern hemisphere. This would subsequently be adjusted into its circular science orbit.[8]
After orbital insertion, MGS performed a series of orbit changes to lower the periapsis of its orbit into the upper fringes of the Martian atmosphere at an altitude of about 110 km (68 mi).[18] During every atmospheric pass, the spacecraft slowed down because of atmospheric resistance. This slowing caused the spacecraft to lose altitude on its next pass through the orbit’s apoapsis. MGS had planned to use this aerobraking technique over a period of four months to lower the high point of its orbit from 54,000 km (33,554 mi) to altitudes near 450 km (280 mi).
About one month into the mission, it was discovered that air pressure from the planet’s atmosphere caused one of the spacecraft’s two solar panels to bend backwards. The panel in question had incurred a small amount of damage shortly after launch, the extent of which did not become apparent until subjected to atmospheric forces. MGS had to be raised out of the atmosphere to prevent further damage to the solar panel and a new mission plan had to be developed.[8]
From May to November 1998, aerobraking was temporarily suspended to allow the orbit to drift into the proper position with respect to the Sun and enable optimal use of the solar panels. Although data collection during aerobraking was not in the original mission plan, all science instruments remained functional and acquired vast amounts of data during this “unexpected bonus period of observation”.[8] The team was able to evaluate more information about the atmosphere over a range of times rather than the anticipated fixed times of 0200 and 1400, as well as collect data during three close encounters with Phobos.[17] (wikipedia: Mars Global Surveyor)
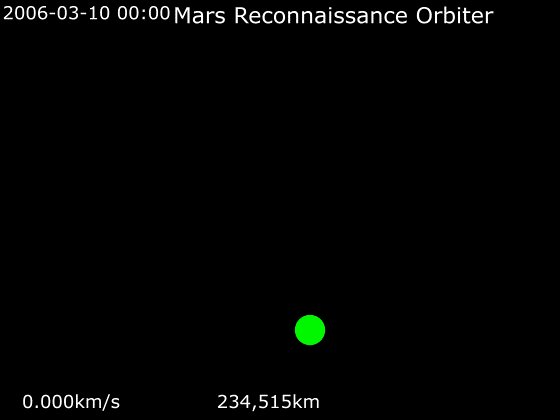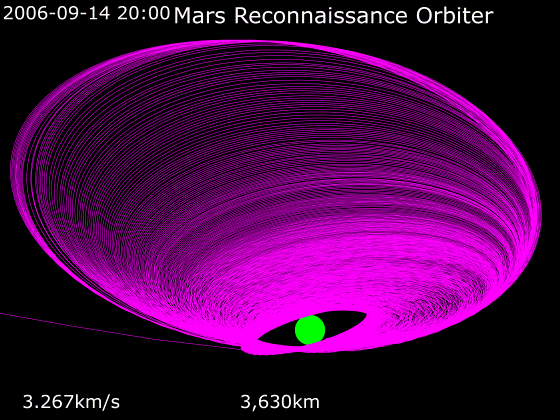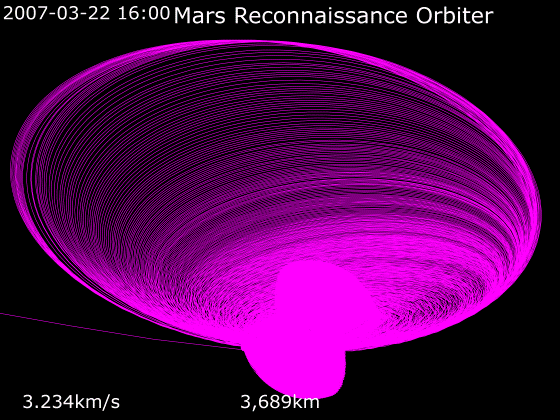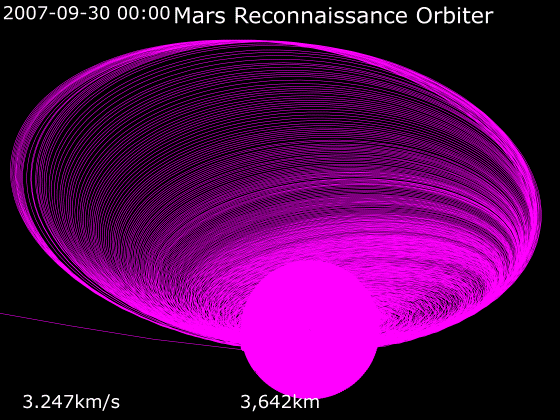
By Phoenix7777 – Own workData source: HORIZONS System, JPL, NASA, CC BY-SA 4.0, Link
This is the maneuver Space X will use to get unmanned spacecraft to Mars. The orbits of the planets have to align for the journey to be possible and this happens about once every 26 months. Each point on the graph represents a window of time. Planetary trajectories evolve over time so you can see how the orbital distance changes in each window and how some missions will be easier than others. Mars has claimed several spacecraft during landing so it will be important to consider all of the variables when charting the surface trajectory as well.
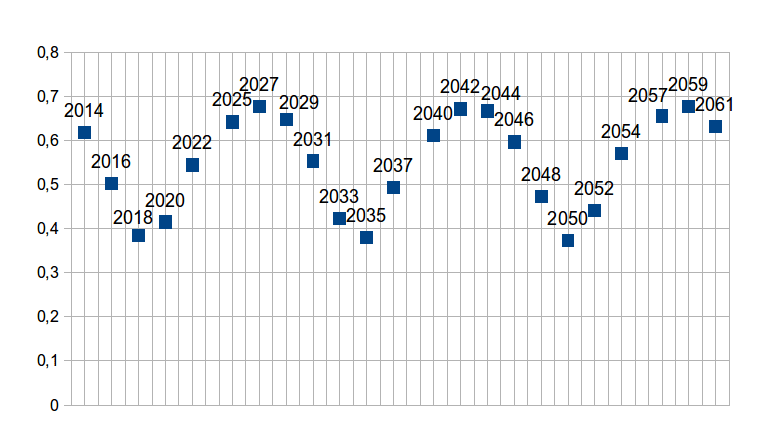
The Length of the Journey to Mars
The duration of the journey to Mars is the hard part. This will be highly influenced by the launch timing due to orbital factors of distance. Perhaps in the near future, we can get the journey time down to a few months, but currently we are looking at 6 to 9 months of time to get to Mars.
The reason for this length of time is that our max speed currently is about 43,000 km/h with an interplanetary spaceship. Starship might achieve 12 km/s to get into a transfer orbit to Mars, but this doesn’t mean it’s constantly accelerating. Once it reaches this speed, it coasts through space, and the speed may change due to gravitational forces.
This is what I want to calculate:
- 1. Analysis on launch date’s effect on the length of the voyage
- 2. Velocity dynamics of the propulsion systems
- 3. The Influence of the Sun over the course of the voyage;
- 4. Relativistic gravity well calculations for the 3 bodies on the spacecraft as velocity changes.
- 5. StarShip, Falcon 9, custom objects 😉 descent into mars’ atmosphere; aero-braking
- 6. Solar assist slingshot maneuver
- 7. Venus assist slingshot maneuver
- 8. Simulate a rocket launch from Texas, (these are notoriously difficult to simulate well and I have only one computer)
- 9. Extra-orbital dynamics; fuel use at various points in the trajectory with low gravity.
- 10. Landing locations; terrain and atmosphere analysis
NASA’s Mars 2020 Mission
In 2020 NASA launched Ingenuity and Perseverance to Mars, two drones to explore the surface of the red planet. Their journey lasted from July 30th to February 18th, totaling 204 days, fairly close to 6 months. You can see the landing to the right; both the use of a heat shield and the landing of the spacecraft into a known crater because of the roughness of the surface and the obvious hazards of the environment, dust, extreme winds, etc. There are more significant hazards to humans in the form of massive blasts of radiation from the sun and even the outer galaxy. Predicting cosmic rays and solar flares will be an absolute necessity to get humans to survive there, not to mention the severe weather.
Pulling the Data together for Simulations
I’m prepping to do some simulation work on this, I’m going to use some computational techniques like parallel GPU computing to try to simulate a spacecraft entering and existing the different atmospheres. Shoulder be fun! Here is the data that I am going to use; directly from NASA. I can not understate the necessity of high quality data for these types of things.
NASA’s Mars Fact Sheet
| Mars | Earth | Ratio (Mars/Earth) | |
|---|---|---|---|
| Mass (1024 kg) | 0.64169 | 5.9722 | 0.107 |
| Volume (1010 km3) | 16.312 | 108.321 | 0.151 |
| Equatorial radius (km) | 3396.2 | 6378.1 | 0.532 |
| Polar radius (km) | 3376.2 | 6356.8 | 0.531 |
| Volumetric mean radius (km) | 3389.5 | 6371.0 | 0.532 |
| Core radius (km) | 1830** | 3485 | 0.525 |
| Ellipticity (Flattening) | 0.00589 | 0.00335 | 1.76 |
| Mean density (kg/m3) | 3934 | 5513 | 0.714 |
| Surface gravity (mean) (m/s2) | 3.73 | 9.82 | 0.380 |
| Surface acceleration (eq) (m/s2) | 3.69 | 9.78 | 0.377 |
| Surface acceleration (pole) (m/s2) | 3.73 | 9.83 | 0.379 |
| Escape velocity (km/s) | 5.03 | 11.19 | 0.450 |
| GM (x 106 km3/s2) | 0.042828 | 0.39860 | 0.107 |
| Bond albedo | 0.250 | 0.294 | 0.850 |
| Geometric albedo | 0.170 | 0.434 | 0.392 |
| V-band magnitude V(1,0) | -1.60 | -3.99 | – |
| Solar irradiance (W/m2) | 586.2 | 1361.0 | 0.431 |
| Black-body temperature (K) | 209.8 | 254.0 | 0.826 |
| Topographic range (km) | 30 | 20 | 1.500 |
| Moment of inertia (I/MR2) | 0.366 | 0.3308 | 1.106 |
| J2 (x 10-6) | 1960.45 | 1082.63 | 1.811 |
| Number of natural satellites | 2 | 1 | |
| Planetary ring system | No | No |
Orbital parameters
| Mars | Earth | Ratio (Mars/Earth) | |
|---|---|---|---|
| Semimajor axis (106 km) | 227.956 | 149.598 | 1.524 |
| Sidereal orbit period (days) | 686.980 | 365.256 | 1.881 |
| Tropical orbit period (days) | 686.973 | 365.242 | 1.881 |
| Perihelion (106 km) | 206.650 | 147.095 | 1.405 |
| Aphelion (106 km) | 249.261 | 152.100 | 1.639 |
| Synodic period (days) | 779.94 | – | – |
| Mean orbital velocity (km/s) | 24.08 | 29.78 | 0.809 |
| Max. orbital velocity (km/s) | 26.50 | 30.29 | 0.875 |
| Min. orbital velocity (km/s) | 21.97 | 29.29 | 0.750 |
| Orbit inclination (deg) | 1.848 | 0.000 | – |
| Orbit eccentricity | 0.0935 | 0.0167 | 5.599 |
| Sidereal rotation period (hrs) | 24.6229 | 23.9345 | 1.029 |
| Length of day (hrs) | 24.6597 | 24.0000 | 1.027 |
| Obliquity to orbit (deg) | 25.19 | 23.44 | 1.075 |
| Inclination of equator (deg) | 25.19 | 23.44 | 1.075 |
Martian Atmosphere
Surface pressure: 6.36 mb at mean radius (variable from 4.0 to 8.7 mb depending on season) [6.9 mb to 9 mb (Viking 1 Lander site)]
Surface density: ~0.020 kg/m3 Scale height: 11.1 km
Total mass of atmosphere: ~2.5 x 1016 kg Average temperature: ~210 K (-63 C)
Diurnal temperature range: 184 K to 242 K (-89 to -31 C)
(Viking 1 Lander site) Wind speeds: 2-7 m/s (summer), 5-10 m/s (fall), 17-30 m/s (38-67mph winter dust storms)
(Viking Lander sites) Mean molecular weight: 43.34
Atmospheric composition (by volume): Major :
Carbon Dioxide (CO2) – 95.1% ;
Nitrogen (N2) – 2.59%;
Argon (Ar) – 1.94%;
Oxygen (O2) – 0.16%;
Carbon Monoxide (CO) – 0.06%
Minor (ppm): Water (H2O) – 210; Nitrogen Oxide (NO) – 100; Neon (Ne) – 2.5; Hydrogen-Deuterium-Oxygen (HDO) – 0.85; Krypton (Kr) – 0.3; Xenon (Xe) – 0.08
NASA’s presence in Space
The next NASA missions planned until Rosalind Franklin Rover (ExoMars) in 2028 (ESA/Roscosmos) which is delayed due to issues with launch partnerships. This mission aims to drill into the surface and search for signs of life…


